Historical Surveying Instruments – Communications of the ACM

Early on, mathematics was of great importance for astronomy, navigation, time measurement, and surveying. As can be seen from contemporary textbooks by Benjamin Bramer, Levinus Hulsius, and Leonhard Zubler, the main focus in the 17th century was on measuring distances and heights. The “geometrical instruments” resembled sectors, and some had a crossbar and a semicircular protractor. Some surveying instruments have survived in museums.
In the third part of his work Apollonius Cattus, Benjamin Bramer speaks of the “geometrical triangulation instrument” that makes it possible to measure heights, depths, lengths, and widths. He dedicated his work to Landgrave Wilhelm von Hessen. The distance meter looks like a sector with two legs, an additional folding rule and sights (see Fig. 1). Sometimes, as shown in his Trigonometria planorum mechanica, it is combined with a protractor and placed on a tripod. It was used to determine the height of towers (see Fig. 2), the depth of wells, and the position of clouds (see Fig. 3). The tool was also suitable for determining the area of polygons (see Fig. 4). Plumb bobs also were used for vertical shafts in a mine. Surveying often was utilized in the service of war. Sectors were each provided with numerous scales. Bramer was the brother-in-law of Jost Bürgi, who created the logarithms independently of John Napier.
Credit: Benjamin Bramer, Apollonius Cattus, Part 3 (Chapter 4)

Credit: Benjamin Bramer, Apollonius Cattus, Part 3 (Chapter 10)

Credit: Benjamin Bramer, Apollonius Cattus, Part 3 (Chapter 17)
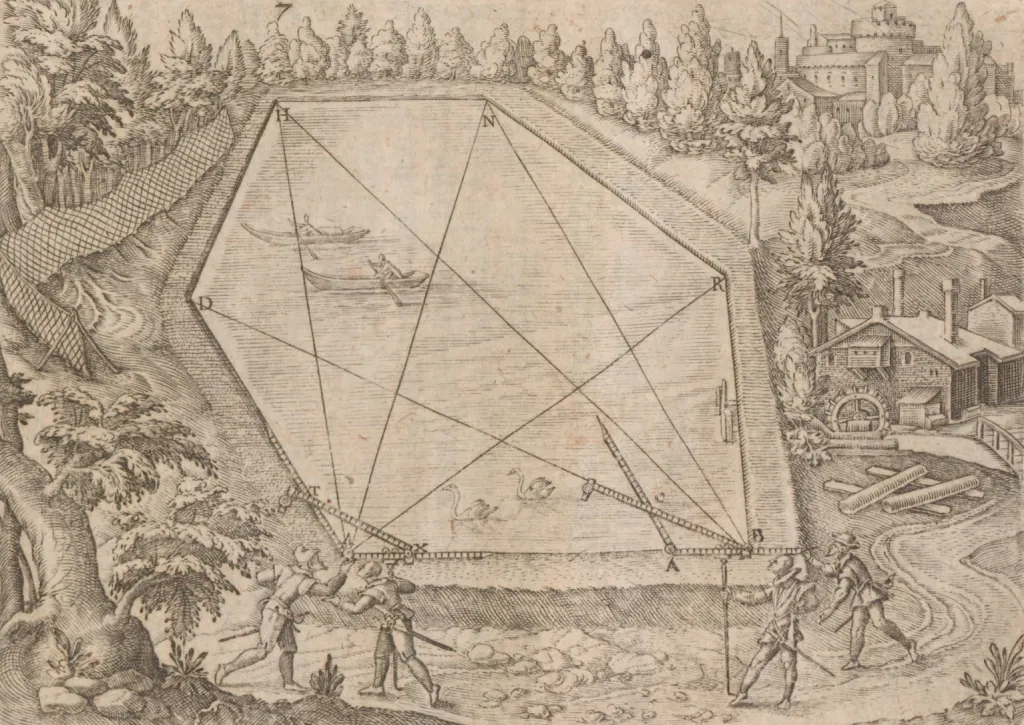
Credit: Benjamin Bramer, Apollonius Cattus, Part 3 (Chapter 5)
Leonhard Zubler from Zurich dedicated his work Novum Instrumentrum Geometricum to Duke Friedrich von Württemberg. He presents his “geometrical instrument,” which consists of a sector with hinged folding rule and a semicircular protractor (semicircular disc with compass) (see Fig. 5). There are also military applications in his textbook (see Fig. 6). Other examples include measuring the height of towers (Fig. 7) or determining the height of mountains. Zubler explains that Thales of Miletus is said to have invented this “geometria” in Egypt and brought it to Greece. He also gives instructions on how to build the triangulation instrument.

Credit: Leonhard Zubler: Novum instrumentum geometricum (page 18)

Credit: Leonhard Zubler: Novum instrumentum geometricum (page 16)

Credit: Leonhard Zubler: Novum instrumentum geometricum (page 25)
In Theoria et Praxis Quadrantis Geometrici, Levinus Hulsius introduces the use of the geometrical quadrant (see Fig. 8). It can be used to determine the height of towers, columns, and trees, for example (see Fig. 9). Distances and the width of rivers can also be calculated. The instrument was also utilized to compute the length of shadows. For comparison: The back of astrolabes (two-dimensional models of the sky) often have a shadow square. This can be used to measure the height of mountains, for example. The term “umbra recta” means straight shadow, while “umbra versa” stands for the reversed shadow. In his work, Hulsius also describes a mathematical device with a compass.

Credit: Levinus Hulsius: Theoria Et Praxis Quadrantis Geometrici (page 9)
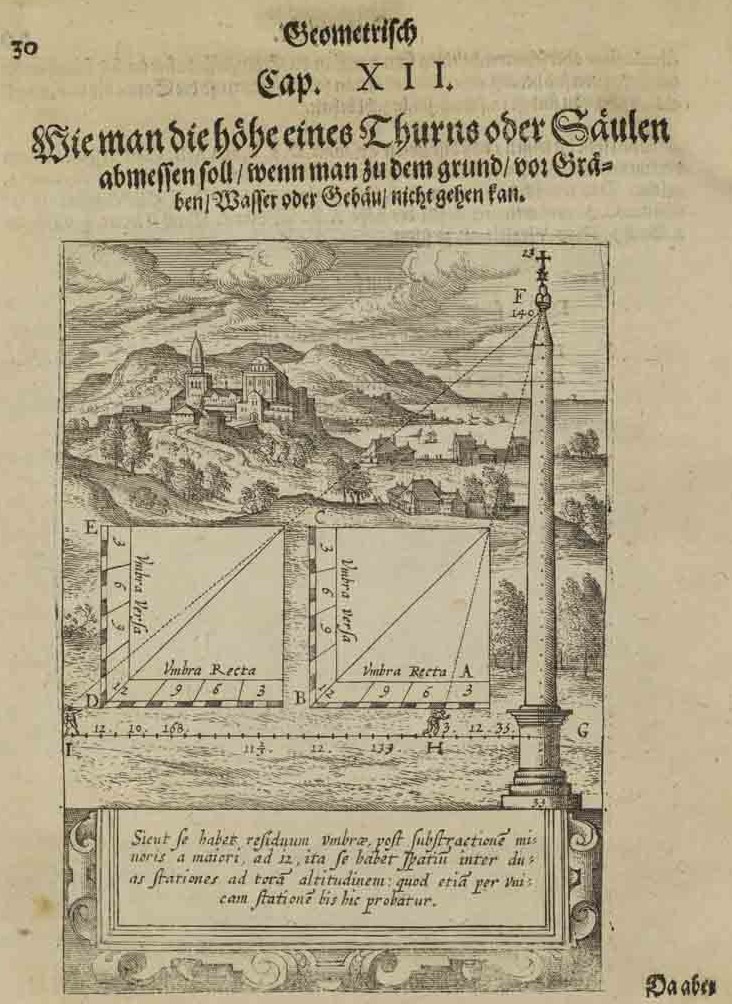
Credit: Levinus Hulsius: Theoria Et Praxis Quadrantis Geometrici (page 30)
Acknowledgments
Many thanks to the libraries for providing high-quality photos.
References
Bramer, B., Trigonometria planorum mechanica oder Underricht unnd Beschreibung eines neuwen und sehr bequemen geometrischen Instruments zu allerhand Abmessung und Solvirung der planischen Triangel derogleich, Paul Egenolff, Marburg 1617, 104 pp., ETH-Bibliothek Zürich, Rar 4012, https://doi.org/10.3931/e-rara-1466, Public Domain Mark
Bramer, B., Apollonius Cattus, Oder Kern der gantzen Geometrieæ In drey Theil, Johann Ingebrands Buchhandlung, Kassel 1684, 272 pages, ETH-Bibliothek Zürich, Rar 5089, https://doi.org/10.3931/e-rara-4043, Public Domain Mark
Hulsius, L., Theoria Et Praxis Quadrantis Geometrici &c., Nürnberg 1594, 74 pp., Digitale Sammlungen der Staatsbibliothek Bamberg, Staatsbibliothek Bamberg, https://nbn-resolving.org/urn:nbn:de:bvb:22-dtl-0000000012, CC BY-SA 4.0
Zubler, L., Novum instrumentum geometricum, Ludwig König, Basel 1625, 75 pp., Universitätsbibliothek Basel, UBH Rb 2067, https://doi.org/10.3931/e-rara-74723, Public Domain Mark
Further reading
Bruderer, H., Meilensteine der Rechentechnik, De Gruyter Oldenbourg, Berlin/Boston, 3rd edition 2020, volume 1, 970 pages, 577 figures, 114 tables, https://doi.org/10.1515/9783110669664
Bruderer, H., Meilensteine der Rechentechnik, De Gruyter Oldenbourg, Berlin/Boston, 3rd edition 2020, volume 2, 1055 pages, 138 figures, 37 tables, https://doi.org/10.1515/9783110669671
Bruderer, H., Milestones in Analog and Digital Computing, Springer Nature Switzerland AG, Cham, 3rd edition 2020, 2 volumes, 2113 pages, 715 illustrations, 151 tables, translated from the German by Dr John McMinn, https://doi.org/10.1007/978-3-030-40974-6
This post is the eighth in a series that presents selected technical marvels from the fields of computing technology, mathematics, astronomy, surveying, time measurement, looms, and automatons (automaton figures, chess-playing machines, musical automatons, automaton writers, drawing automatons, automaton clocks, picture clocks, globes, and historical robots). The examples are largely from the 15th to 20th centuries and do not claim to be exhaustive. In particular, artifacts are included for which high-quality illustrations are available. Most of the objects come from Europe, with a few from Africa, America, Asia, and Australia. The order is predominantly chronological. Famous names such as Archimedes, Hipparchus, Heron of Alexandria, Leonardo da Vinci, Galileo Galilei, John Napier, Jost Bürgi, Blaise Pascal, Gottfried Wilhelm Leibniz, and Charles Babbage are associated with these works. In some cases, however, the inventors are unknown (e.g. abacus, Antikythera Mechanism, yupana, quipu); see Technical Marvels, Part 7: Musical Clocks and Organs – Communications of the ACM.

Herbert Bruderer is a retired lecturer in the Department of Computer Science at ETH Zurich and a historian of technology. He recently was added to the Honor Roll of the IT History Society.




